Dynamic Ladas Super Surface helps optimize and refine IOL calculations
Each data point can reshape the Ladas Super Surface, which leads to more accurate results from the Ladas Super Formula.
Historically, we have been advised to optimize specific IOL formulae for our patients in order to better predict the postoperative refraction and to deliver better visual outcomes. This means that we need to analyze our patient data and then make adjustments so that future IOL calculations are more accurate.
The idea is that there are differences in calibration of the devices used in biometry and even differences in surgical techniques that can influence the IOL power calculation and thus the postoperative refractive result. This makes sense because if one keratometer reads 0.5 D different than another, there may be about a 0.5 D difference in IOL power if all other factors are equal. Surgical technique typically refers to steps that affect the effective lens position such as the overlap of the capsulorrhexis over the optic edge in order to secure it. A large, non-overlapping capsulorrhexis may allow the IOL optic to come forward, thereby resulting in a more myopic outcome.
Optimizing the A-constant for the SRK/T formula, the “Surgeon Factor” for the Holladay formula, the anterior chamber depth for the Hoffer Q, or the a0/a1/a2 for the Haigis will affect the IOL power evenly across the board, from the most hyperopic eye to the most myopic one. This optimization will help to some degree, but each IOL formula tends to have a “sweet spot” at which it is most accurate and also areas at which it is less precise. What we actually need to do, in order to improve accuracy, is optimize the formula across the spectrum and with all known variables.
It has been said that at least 20 eyes need to be considered to optimize IOL calculations with a particular formula. But what about comparing one formula with another? Do we need to go back and calculate scores of eyes in order to improve accuracy? Probably not because we would be better served to compare the two formulae and determine at which parameters there is a discrepancy between them. The Ladas-Siddiqui graph (Figure 1) is a way of comparing two formulae across a range of biometric parameters. The area of clinical agreement can be identified as well as the area of discrepancy. For the best results, we should focus our efforts on the area of clinical disagreement because this is likely to yield the most benefit.
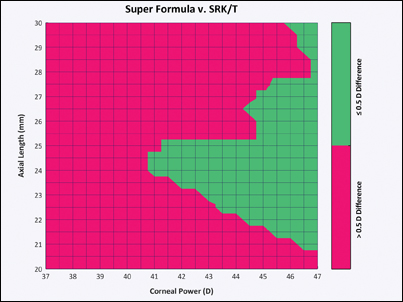
Images: Devgan U
The variables that determine IOL power are finite. These include the corneal power, both anterior and posterior, the axial length of the eye, and the position where the IOL will ultimately sit, which is called the effective lens position. The calculation of the effective lens position is the most challenging, and many factors are thought to influence it, such as keratometry, axial length, white-to-white, anterior chamber depth, refraction, age and even gender. The geometry and design of the IOL also affect the IOL power calculation. All known variables are intimately related and cannot be teased out one by one without a more involved mathematical and graphical solution.
The Ladas Super Formula is an amalgamation of existing IOL formulae that seeks to amplify the accuracy while minimizing the refractive misses. By plotting out the IOL calculations in three dimensions, we can see that it is a complex shape that changes curvature depending on the eye parameters. Each block of the graph (Figure 2) can be optimized until a continuum is generated. Other variables can be embedded into the Ladas Super Surface appropriately at specific regions. For example, if anterior chamber depth is a factor at short axial lengths, then it can be embedded in that region. The caveat is that a variable cannot be added without it affecting the other factors that are already being accounted for. Other parameters can be continuously evaluated to see if they help refine the Ladas Super Surface, such as posterior corneal power, which is currently not used in formulae.
The goal is to have a fluid, dynamic Ladas Super Surface whereby we can optimize the lens calculations for an entire range of eyes for the cohort of all cataract surgeons or for each individual surgeon. Every time a data point of actual outcome data is entered, it can help to more accurately reshape the Ladas Super Surface and thereby give more accurate results from the Ladas Super Formula.
- For more information:
- Uday Devgan, MD, is in private practice at Devgan Eye Surgery, Chief of Ophthalmology at Olive View UCLA Medical Center and Clinical Professor of Ophthalmology at the Jules Stein Eye Institute, UCLA School of Medicine. He can be reached at 11600 Wilshire Blvd. #200, Los Angeles, CA 90025; email: devgan@gmail.com; website: www.DevganEye.com.
Disclosure: Devgan reports no relevant financial disclosures. He reports he is working with Drs. Ladas, Siddiqui and Jun to further improve IOL calculations.
