‘Super Surface’ and ‘Super Formula’ concepts increase IOL calculation accuracy
Click Here to Manage Email Alerts
IOL power calculations for cataract surgery have come a long way since the days of empiric regression formulae such as the SRK and SRK II. We now tend to use theoretical methods such as the Holladay, SRK/T, Hoffer Q and Haigis, among others. While we think of these formulae as two-dimensional entities, there is a benefit of plotting them in three dimensions.
John Ladas, MD, PhD, had an idea: Could a graphical solution be used to solve the complex mathematical problem of IOL power calculation? The concept that he came up with is a novel method of characterizing and conceptualizing IOL formulae in three dimensions to ease interpretation and visualization. Current formulae such as the Holladay, Haigis, Hoffer and Sanders/Retzlaff/Kraff are very sophisticated and can be conceptualized better when seen in this manner. This method makes it easy to compare and analyze one formula vs. another or even all formulae at once.
Working with Aazim Siddiqui, MD, this concept was made into a reality when all IOL formulas were rendered as 3-D surfaces. An example of this can be seen in Figure 1, in which we can see the 3-D plotting of the SRK/T, Hoffer Q and the two together. We have learned from experience that certain methods are more accurate than others for different types of eyes. Retrospective analyses have shown that while the SRK/T tends to be better for longer eyes, very short eyes may do better with the Hoffer Q. In Figure 1c, the comparison shows that the Hoffer Q and SRK/T differ as we change the specific input parameters of axial length and corneal power.
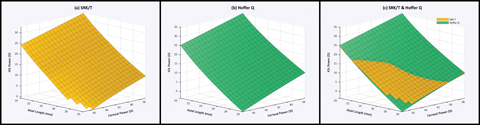
Images: Devgan U
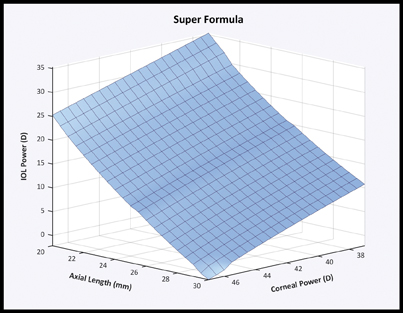
This concept of 3-D graphical analysis ultimately led to the creation of the IOL “Super Formula,” which was derived from a singular “Super Surface” that amalgamated the best portions from many of the modern IOL formulas. The Super Formula represents the strengths of all incorporated formulae and avoids their respective weaknesses. A single Super Formula can bring together all existing IOL calculation methods into one seamless result that is more accurate than any single formula.
The concept of using a Super Surface can also allow for targeted optimization of individual groups, formulae or lens types. In addition, another advantage of approaching the problem in this way is that the Super Surface and thus the Super Formula are “living” and can evolve. This is due to the power of the Ladas-Siddiqui graph, which allows for precise comparisons among this formula, all other formulas in existence and even future formulas. Other variables such as anterior chamber depth can also be integrated, and accuracy can be further improved.
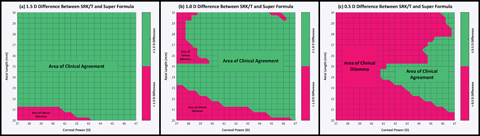
We can compare any formula to the Super Formula at various corneal powers and axial lengths by plotting them together in a Ladas-Siddiqui graph. For example, we can look at the SRK/T formula results and how they differ from the Super Formula results at tolerances of 0.5 D, 1 D and 1.5 D. The areas of clinical dilemma are where there are differences, and the areas of agreement are where the results are similar. Because postoperative differences of 0.5 D are small, we can focus our efforts at the areas where there is a 1 D or 1.5 D disparity threshold to save time and resources.
Ladas and Siddiqui have created both a unique way of looking at the IOL calculation data using the Super Surface and a Super Formula that uses the ideal portions and strengths of the existing IOL calculation formulae. Any future formula or method of lens power calculation can be compared with and incorporated into the Super Formula, such as the Wang-Koch axial length modification for highly myopic eyes.
The ultimate benefit of the Super Surface and Super Formula is a higher degree of accuracy in our IOL power calculations for cataract surgery patients. In an article published in JAMA Ophthalmology, the Super Formula managed to localize to the most appropriate IOL power 100% of the time, which was deemed to be in line with best practices and potentially solve a clinical dilemma for the surgeon.
- Reference:
- Ladas JG, et al. JAMA Ophthalmol. 2015;doi:10:1001/jamaophthalmol.2015.3832.
- For more information:
- Uday Devgan, MD, is in private practice at Devgan Eye Surgery, Chief of Ophthalmology at Olive View UCLA Medical Center and Clinical Professor of Ophthalmology at the Jules Stein Eye Institute, UCLA School of Medicine. He can be reached at 11600 Wilshire Blvd. #200, Los Angeles, CA 90025; email: devgan@gmail.com; website: www.DevganEye.com.
Disclosure: Devgan reports no relevant financial disclosures.
