Anterior chamber depth plays critical role in IOL calculations
The anterior chamber depth is directly related to the effective lens position.
Essentially all formulae for calculating IOL power for cataract surgery have the same structure: a vergence calculation and a way of determining the anterior chamber depth and the final resting position of the optic. This effective lens position, or ELP, is the principal way that the formulae differ, with the vergence calculation being essentially identical across the board. The anterior chamber depth, or ACD, relates directly to the ELP and therefore has a critical role in the accuracy of the IOL calculations.
Jack T. Holladay, MD, MSEE, FACS, has taught us that eyes can have a shallow, normal or deep anterior chamber in the setting of a short, average or long axial length. These various combinations can have large differences in lens calculations. For example, a small anterior chamber in a short eye is the typical nanophthalmic eye in which we can expect the IOL power to be high. However, an equally short eye with a deep anterior chamber will produce a far larger IOL power and more uncertainty in the IOL power calculation because the ELP will be farther from the cornea and closer to the retina.
Using concepts developed by John Ladas, MD, PhD, we can make three-dimensional graphs of these concepts to help us better understand IOL calculations. In previous columns, we have looked at the Ladas Super Formula and Ladas Super Surface, which plot the keratometry, axial length and IOL power in three dimensions. The concept of separating the ACD, and therefore the ELP, calculation from the vergence calculation can be helpful in many different situations. And as with the Ladas Super Formula, this data can evolve.
For post-refractive IOL calculations, what is needed is a way to accurately measure the anterior and posterior power of the cornea and then to combine that value with the ACD calculation. This will result in an accurate IOL power calculation without having to change the vergence calculations. For intraoperative aberrometry, the derived IOL power determination is most dependent on the ability to predict the final IOL position (ELP), and that is the unknown variable. For accommodating IOLs, the effectiveness is dependent on the ACD, the IOL power and the axial length. For ray tracing as used to determine IOL power, the unknown variable is the ACD. Even for our current IOLs, which have a variable lens geometry across their power range, the ELP changes as the principal plane of the optic changes.
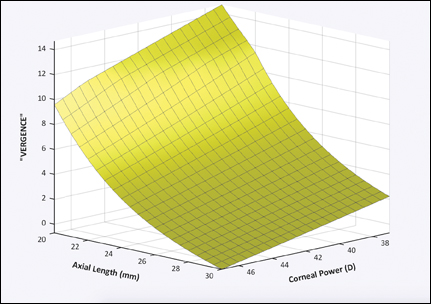
Images: Ladas J
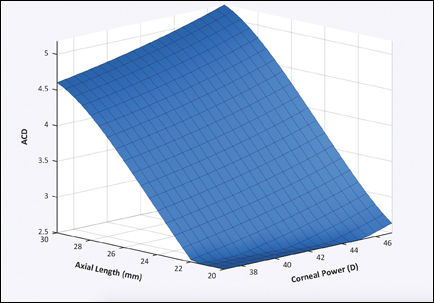
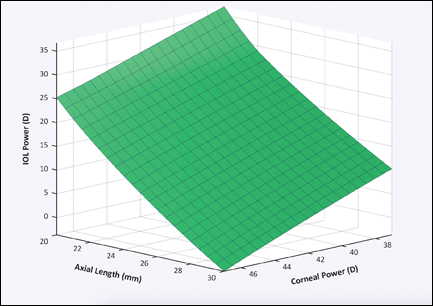
Clinical scenarios
The eyes that have had prior corneal refractive surgery pose a challenge for IOL calculations because of two main issues: The determination of the true corneal power tends to be inaccurate, and the portion of the formulae used for ACD determination is fooled by the unusual corneal power. The Aramberri double-K method uses a fixed value of 43.8 D for the corneal power for the ACD/ELP determination and then uses the actual post-refractive surgery K value for the vergence calculation. This is a method of doing exactly what is proposed here: splitting the ACD calculation from the vergence calculation. We also realize that no matter how flat the cornea is from the prior refractive surgery, there will be a lower limit to the anterior chamber depth.
For very large eyes with large axial lengths, we expect to have a deeper anterior chamber depth and the ELP will be more posterior in the eye. But here, too, there is a limit because even eyes with axial lengths of 30 mm do not have ACDs beyond a certain level. Yes, we can have deep anterior chambers, but there seems to be a limit.
And as previously stated, the ACD and ELP are the most critical in the very small eyes with the short axial lengths. As the measured ACD gets larger, the optic sits closer to the retina, and as such, a much higher IOL power is needed.
- For more information:
- Uday Devgan, MD, is in private practice at Devgan Eye Surgery, Chief of Ophthalmology at Olive View UCLA Medical Center and Clinical Professor of Ophthalmology at the Jules Stein Eye Institute, UCLA School of Medicine. He can be reached at 11600 Wilshire Blvd. #200, Los Angeles, CA 90025; email: devgan@gmail.com; website: www.DevganEye.com.
Disclosure: Devgan reports no relevant financial disclosures. He reports he is working with Drs. Ladas, Siddiqui and Jun to further improve IOL calculations.
