Which IOL calculation formula should you use for different scenarios?
The Ladas Super Formula and Ladas Super Surface are useful when standard formulae yield different results.
For many years we have been instructed to use theoretical formulae to do our IOL calculations. These formulae include the third-generation Holladay 1, SRK/T and Hoffer Q, as well as the fourth-generation Holladay 2, Haigis, Olsen and Barrett methods. All of these formulae have similarities, but they also have differences and eyes in which they are particularly well suited and eyes in which they are less accurate. There are additional modifications to these formulae, such as the Wang-Koch axial length adjustment for highly myopic eyes, in an effort to improve accuracy. So it begs the question, “When do we use which formula in order to achieve the most accurate results?”
I think that we may have entered a new era of IOL calculations. In my previous column, I wrote about the concept of a “Super Surface” and a “Super Formula” as published by John Ladas, MD, PhD, and his co-authors. It is an important concept that may change the way that we approach IOL calculations for our cataract surgery patients.
All current IOL calculation formulae and even all future methods can be placed into the Ladas Super Formula and be represented on the Ladas Super Surface. The surface and formula are both dynamic so that they can be further refined and optimized based on any new published studies and even with user data, both individually and as a large group.
You are selecting the IOL powers for your next surgery schedule, and you are confronted with a dilemma: The three third-generation formulae that you have selected each come up with a different IOL power. Should you check the more complex fourth-generation formulae to compare? If the results are different, then which one do you choose? Let’s explore a few different scenarios.
Small eyes with short axial lengths
These eyes are notoriously difficult to calculate with a high degree of accuracy because a very small shift in the effective lens position of the IOL can change the calculations dramatically. We should look carefully at the anterior segment size because whether the anterior chamber is shallow or deep changes the calculation. In published literature, the Hoffer Q is noted to perform reasonably well in these eyes, and fourth generation such as Haigis and Holladay 2, which incorporate anterior chamber depth measurements, can be even more accurate. The Ladas Super Formula would localize your variables on the Ladas Super Surface and would give you the most accurate calculation.
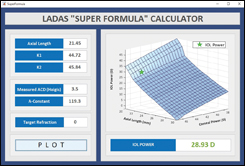
Images: Devgan U
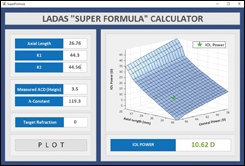

Myopic eyes with long axial lengths
In these eyes with axial lengths greater than 26 mm, the results of our calculations tend to be less accurate unless we remember to use the Wang-Koch adjustment to the axial length before doing the calculation. With the Ladas Super Formula, there is no need to remember because it will be calculated for you automatically. And when further refinements are noted by the authors, they will be updated into the Ladas Super Formula.
Unusual eyes
What about an eye with an axial length of 29 mm and a high keratometry value of 47 D, such as a patient with forme fruste keratoconus? A cursory review of the literature may let one believe that the SRK/T is an appropriate choice in a “longer eye.” However, using the Ladas Super Surface may be a better option because the SRK/T suffers from something called the “null hypothesis” in longer eyes with high keratometry values, which can result in a calculation of a negative square root and an imaginary number. This region would not be included on the Ladas Super Surface, so the surgeon would instead be offered the most accurate IOL power calculation based on other formulae.
These are three scenarios that we have all encountered in which the standard formulae can yield different results. The Ladas Super Formula seeks to combine the best of all formulae while avoiding their respective weaknesses. In addition, the program will tell you when it differs from other formulae, thereby giving the surgeon an opportunity to step back and reassess the situation.
Results from testing a wide range of 100 eyes
In the paper published by Ladas, an initial test of 100 eyes was completed across a wide range of corneal powers and axial lengths. The Super Formula localized to the correct portion of the Super Surface 100% of the time and chose the best IOL power 100% of the time. The individual formulae did not fare as well, with 70% for Hoffer Q, 84% for Holladay 1, 78% for Holladay 1 with Wang-Koch adjustment, 76% for SRK/T and 52% for Haigis.
These initial results are just the beginning because the beauty of the Ladas Super Surface is its ability to evolve. While four formulae with or without adjustment and consideration of lens design were included in the initial iteration, future iterations will continue to get better. It is well known that the best way to improve a formula is with optimization, and this has already started. Future columns will discuss how this is achieved.
- Reference:
- Ladas JG, et al. JAMA Ophthalmol. 2015;doi:10.1001/jamaophthalmol.2015.3832.
- For more information:
- Uday Devgan, MD, is in private practice at Devgan Eye Surgery, Chief of Ophthalmology at Olive View UCLA Medical Center and Clinical Professor of Ophthalmology at the Jules Stein Eye Institute, UCLA School of Medicine. He can be reached at 11600 Wilshire Blvd. #200, Los Angeles, CA 90025; email: devgan@gmail.com; website: www.DevganEye.com.
Disclosure: Devgan reports he is one of the co-authors on the cited Ladas paper, and he is the registrant for the www.IOLcalc.com website.









