Mathematics of planning cataract surgery can ensure more accurate refractive results
Determining the IOL position helps to refine the spherical refractive outcome, and factoring in corneal astigmatism can result in a lower cylindrical error.
Due in part to the physics of optics, mathematics plays a significant role in planning cataract surgery. Understanding the position of the IOL within the eye helps to refine the spherical refractive outcome of the surgery, while analysis and treatment of the corneal astigmatism can result in a lower cylindrical refractive error.
Effect of eye type on spherical IOL power
Jack T. Holladay, MD, MSEE, has done much of the pioneering work for IOL calculations in cataract surgery. Among the biggest challenges is determining exactly where in the eye the IOL will sit after cataract surgery. In my last column, I explained that if the same IOL power is placed in a more anterior position in the eye, there will be a more myopic result. Using the Holladay schema, we can see that with a combination of different axial lengths and anterior segment sizes, there are effectively nine different types of eyes that we need to consider for our lens power calculations (Figure 1). While the more common third-generation lens formulae (Holladay 1, SRK-T and Hoffer Q) work well with normal eyes, the Holladay 2 calculation tends to be more accurate across a broad range of eyes.
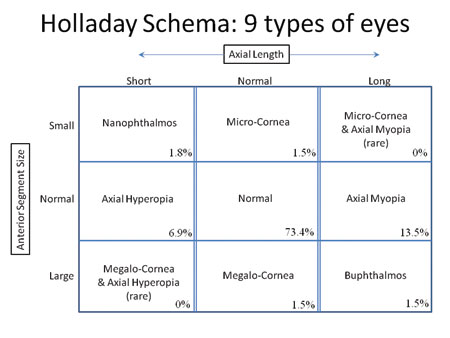
Figure 1. Jack T. Holladay, MD, MSEE, has noted that axial length does not necessarily correlate to the size of the anterior segment, which can result in less accuracy with lens power calculations due to difficulty in determining the effective lens position. The percentage in each cell indicates the percentage of eyes in his study that had those dimensions.
Images: Devgan U
When intracapsular cataract surgery was routinely performed many years ago, the determination of anterior chamber depth helped to ascertain the ideal power for the IOL that was placed in the anterior chamber, directly on the iris. This measured depth was referred to as the anterior chamber depth (ACD), and the name stuck even as we moved toward placing IOLs in the capsular bag, which is in the posterior chamber. When it comes to IOL calculations, the ACD is different from the anatomic true anterior chamber depth. With an IOL placed in the capsular bag, we can get an ACD that measures 5.5 mm or more even though the anatomic anterior chamber depth of the eye may only be 3 or 4 mm.
Due to the confusion regarding ACD, Holladay proposed using the term “effective lens position” instead, and his initial formula, the Holladay 1 equation, uses SF, which stands for surgeon factor. The SRK-T formula uses the term “A-constant” in reference to the effective lens position. Thus ACD, SF and A-constant all measure the same thing, and we can convert between them using simple equations (Figure 2).
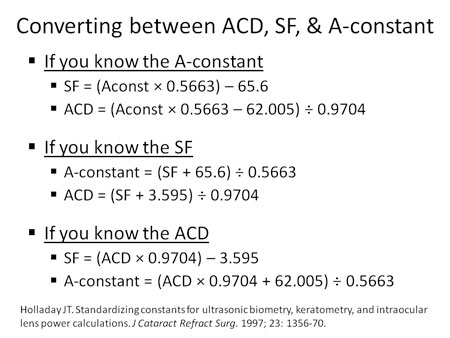
Figure 2. The A-constant, SF (surgeon factor) and ACD (IOL anterior chamber depth) all reflect the effective lens position of the IOL. We can convert between these units using the simple equations published by Holladay.
Factoring in corneal astigmatism
The lens formulae calculate the IOL power for a given postoperative refractive goal. It is important to remember that this is the spherical equivalent of that goal. If the patient has 2 D of corneal astigmatism steep at 90°, then the predicted power of plano with a specific spherical IOL is really –1.00 +2.00 × 90°, which can also be represented as +1.00 –2.00 × 180° (Figure 3).
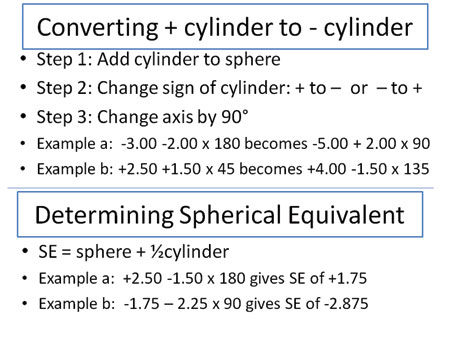
Figure 3. Converting positive cylinder to negative cylinder in a refraction can be done in three simple steps. Determining the spherical equivalent of a refraction is even easier and can be accomplished by simply adding half of the cylinder to the sphere.
To increase the accuracy of the cylinder component of the postoperative refraction, we need to take into account the corneal astigmatism. The total astigmatism present in the patient’s refraction is a combination of the corneal astigmatism and the lenticular astigmatism present in the crystalline lens. Because we are removing the crystalline lens with our cataract surgery, we are primarily interested in the corneal astigmatism for our calculations. We can effectively treat this corneal astigmatism at the time of cataract surgery by placing relaxing incisions on the steep corneal axis or by implanting a toric IOL. In either case we will need to make a phaco incision for the surgery, and that too will have an astigmatic effect.
Calculating the surgically induced astigmatism (SIA) from our routine phaco incision is an important step in honing our refractive results. If a patient has a cornea that is steeper at 90° by 0.5 D and we place our phaco incision, which causes an SIA of 0.4 D of flattening, at the usual temporal position (180°), then the patient will end up with 0.9 D of postop corneal astigmatism steep at 90°. If, however, we had placed the phaco incision on the steep corneal axis of 90°, then it would have addressed the pre-existing astigmatism and resulted in an eye with 0.1 D of postop corneal astigmatism steep at 90°.
If we know the preop keratometry, postop keratometry and position of the incision, we can use vector math to determine the SIA of the incision. Warren Hill, MD, has an excellent guide as well as an online SIA calculator that will do the mathematical calculations for you at www.doctor-hill.com, and ophthalmologists can input data from their own cases. Once the SIA is known, we can incorporate that into our surgical planning for corneal relaxing incisions or toric IOLs.
By taking into consideration the individual ocular anatomy, converting values for effective lens position to the appropriate units for our formulae and factoring in the corneal astigmatism, we can produce more accurate postop refractive results for our patients.
References:
- Hill WE. The surgically induced astigmatism calculator. Cataract & Refractive Surgery Today. 2008;2:44-48.
- Holladay JT. Standardizing constants for ultrasonic biometry, keratometry, and intraocular lens power calculations. J Cataract Refract Surg. 1997;23(9):1356-1370.
For more information:
- Uday Devgan, MD, is in private practice at Devgan Eye Surgery in Los Angeles and Beverly Hills. He can be reached at 11600 Wilshire Blvd., Suite 200, Los Angeles, CA 90025; 800-337-1969; email: devgan@gmail.com; website: www.DevganEye.com.
- Disclosure: Devgan has no relevant financial disclosures.








