The Physics of Light

Introduction

Vision is the perception of light. To comprehend the nature of human vision, an understanding of the properties of light is necessary. Many of the technologies used for examining the eye and treating ocular disease take advantage of the properties of light to better enable clinicians to perform successful evaluations. For example, the slit lamp uses electricity to generate light and lenses to project light into the eye. It uses more lenses to provide the viewer with a magnified image of the patient’s eye, and takes advantage of scatter to help visualize the cornea and crystalline lens, and their respective clarities. This tutorial specifically describes where light comes from, how it interacts with objects, and how can it be used to aid diagnosis and treatment of eye disorders.

Let there be Light

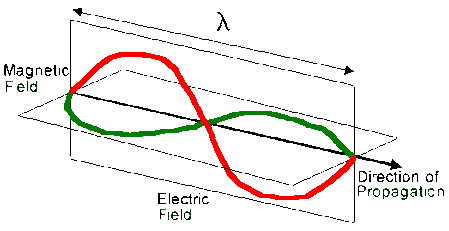
In the classical view of an atom, there is a nucleus with a series of electrons orbiting about it. Typically atoms are in the resting state, which means that the negatively charged electron cloud is in a harmonious balance with its positively charge nucleus. Excited atoms have electrons that have been forced into a higher orbit or energy level. Excited atoms are out of balance and are driven to return to their resting state. In order to do so, excited atoms must give up energy. This energy is released in the form of a photon. A photon is a packet of energy that can propagate through space until it interacts with another object. The photon propagates through space in the form of an electromagnetic wave. Electromagnetic waves have an electric field and a magnetic field, which oscillate as the waves move through space. The electric and magnetic fields vary within planes that are perpendicular to each other, and also perpendicular to the direction in which the wave is traveling. Slide 1 shows a depiction of an electromagnetic wave.

Slide 1. Electromagnetic field.

As with all waves, there is a distance between the crests of the waves, known as the wavelength, or l. The wavelength is inversely proportional to the amount of energy the atom gave up. Thus, photons with a short wavelength have high energy and photons with long wavelengths have lower energy. Photons will travel through vacuum at a constant speed. This is called the speed of light, c, and is equal to 300,000,000 meters per second. As photons enter media other than vacuum, they will slow down. The index of refraction, n, of a given media is the ratio of the speed of light in vacuum to the speed of light within the media.

One final concept that is useful in describing photons and electromagnetic waves is frequency, n. The frequency is the number of oscillations per second the electromagnetic wave goes through at a given point in space. The frequency is directly proportional to the energy given up by the atom. Thus, high frequency light has high energy and low frequency light carries lower energy. Frequency is also related to the wavelength of light and is given by n = c / l.

A useful analogy for understanding these concepts is wading into the ocean. As the water waves come into shore, they will strike the wader. The distance between the crests of the waves is the wavelength. How fast the waves come into the shore is the speed of the wave, and how frequently the wader is struck is the frequency of the waves.¹

Waves versus Photons

In classical physics, wave phenomena such as sound and water waves exhibit certain physical properties and discrete particles such as baseballs and sand grains exhibit different physical properties. As we move into the quantum world, however, the distinction between waves and particles begins to blur. Photons are discrete quantum particles that exhibit wave-like properties. A full description of these quantum effects is well beyond the scope of this tutorial. For the purposes of this tutorial, light will be considered a wave when dealing with macroscopic entities and as a particle when dealing with atomic or molecular entities.¹

The Electromagnetic Spectrum

The amount of energy an excited atom gives off determines the wavelength of the photons that it emits. There is a continuum of wavelengths possible and this continuum is known as the electromagnetic spectrum. The shortest wavelengths possible come from gamma rays, which are associated with very violent cosmic events. The wavelengths for gamma rays are smaller than the atomic dimension. The next smallest on the wavelength scale are X-rays. Further along the electromagnetic spectrum is ultraviolet and then visible light. Photons with wavelengths longer than the visible spectrum are infrared. Finally, radio waves make up the longest wavelengths of the spectrum. Each of these individual portions of the spectrum is used for different purposes that depend on their ability to propagate through different media and their energy. For example, X-rays are used to image internal structures in the body, because the rays can penetrate and propagate through biological tissue. Radio waves are used to broadcast radio and television programming because they propagate well through the atmosphere.²

Light and Color

To this point, "light" has been used loosely. Technically, light is only electromagnetic waves that fall within the visible spectrum. In other words, light corresponds to photons that can be detected by the human visual system. Photons falling outside of the visible spectrum are technically not light, although the terms ultraviolet light and infrared light are often used. The term visible light is also commonly used, but it is redundant. Light corresponds to wavelengths from approximately 380 nm to 780 nm, with the shorter wavelengths being perceived as the blue end of the spectrum and the longer wavelengths evoking the perception of the red end of the spectrum. If a person is presented with individual wavelengths ranging from 380 nm to 780 nm, he or she will perceive all the possible colors found in the spectrum. Most light sources, however, do not emit a single wavelength, but simultaneously emit photons of many different wavelengths. Photoreceptors in the retina absorb these photons and convert them into a signal we can perceive.

There are three types of photoreceptors associated with color vision. These are known as the L- (long), M- (middle) and S- (short) cones. The cones control our color vision. The long, middle and short monikers refer to the wavelengths of the visible spectrum. Thus, the S-cones respond predominantly to the blue end of the spectrum. The M-cones respond to the middle, or green, portion of the spectrum, while the L-cones respond to the long wavelengths or the red end of the spectrum. Two light sources can emit drastically different spectrums of photons, yet still appear to be the same color. Although different wavelengths of light for the two sources are entering the eye, the absorption of the photons by the various types of cone receptors can occur in the same proportions. In this manner, the signals sent to the visual cortex from the two light sources are identical. The two light sources in this case are known as metamers. This effect means that the light itself is not colored, but that color is a property of the way the visual system detects light.³

Slide 2. Constructive and destructive interference.

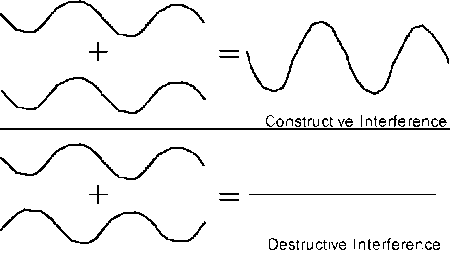
One property of wave phenomenon is interference. Interference is the addition of two waves to form a new wave. In places where the crest of one wave meets the crest of a second wave, the crests combine to form an even larger peak in the wave. In places where the trough of one wave meets the trough of a second wave, the waves combine to form an even deeper depression. In places where the crest of one wave meets the trough of another wave, the two cancel each other’s effects and there is no peak or trough in the resulting wave. When two waves combine to form a wave with larger amplitude, it is called constructive interference. In cases where the two waves cancel each other, the process is called destructive interference.

An example of this phenomenon in audio is noise-canceling headphones. The offending noise is analyzed and a second sound wave is generated to cause destructive interference and effectively eliminate the noise from being heard. In optics, interference is routinely used to measure the quality and shape of optical surfaces and to bypass the optics of the eye and project contrast sensitivity fringes directly onto the retina. Interference is also seen with laser sources. When a laser spot is shone onto a rough surface, the spot appears granular due to speckle. Speckle is due to the random interference of the laser light. The dark speckles correspond to regions of destructive interference and the bright speckles correspond to regions of constructive interference. Slide 2 illustrates constructive and destructive interference.⁴

Coherence

Not all light will interfere. Coherence is a description of the ability of two waves to interfere. Incoherent light will not interact to have constructive or destructive interference. Conversely, coherent light will demonstrate these effects. Generally, two coherent waves must have approximately the same wavelength, come from the same source, and have left the source at approximately the same time. Lasers are highly coherent and incandescent and fluorescent lights are higher incoherent.⁴

Diffraction

Slide 3. Diffraction.

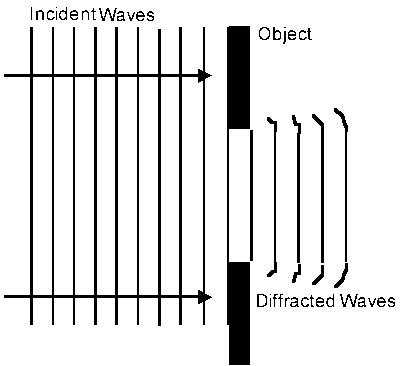
A second property of wave phenomena is diffraction. Whereas interference is one wave interacting with another wave, diffraction is a wave interacting with an object in the environment. As the wave passes close to atoms of an object, the light and atoms interact with one another. This interaction causes the wave to become distorted. If the distorted wave is incident upon a surface, a diffraction pattern will be seen.

Diffraction can be seen by viewing a distant street lamp through a window screen. As the light leaves the street lamp, it freely propagates through the atmosphere. In passing through the screen, the wave front interacts with the atoms making up the screen mesh and the wave becomes distorted. In viewing the street lamp through the screen, the diffraction pattern is projected onto the retina. The diffraction pattern in this case appears to be a cross. As the light passed through the screen, the horizontal fibers of the mesh caused light to diffract in the up and down direction, while the vertical fibers causes light to diffract left and right. The resulting diffraction pattern is a superposition of the two effects, which appears as a cross. Diffraction causes the boundaries of shadows to be soft. Diffraction also limits how small a spot can be placed onto the retina. As light enters the eye, it interacts with the iris. When viewing a distant point source, if the optics of the eye were perfect, the diffraction pattern caused by the pupil would be seen. Thus, diffraction ultimately limits our acuity because it forces a point of light to have a finite size in the retina. Generally, the smaller the area that the wave is forced through, the larger the diffraction pattern will be. Slide 3 illustrates diffraction.⁴

Slide 4. Scatter.

Scatter

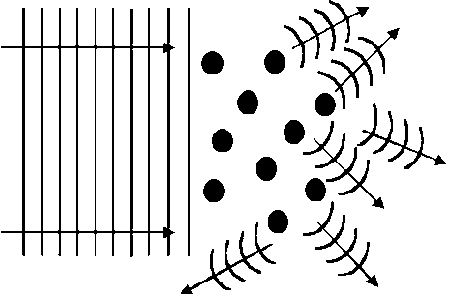
Scatter is another diffraction effect in which light interacts with a series of small particles. The particles absorb the light and re-radiate it into all different directions. The size and spacing between particles determine the degree of scatter. Furthermore, shorter wavelengths are more prone to scattering than longer wavelengths. Smoke, fog, corneal edema, and cataracts all cause scatter. As smoke scatters light, it takes on a blue tinge due to the higher degree of scatter in the blue end of the spectrum. Since infrared light is less prone to scatter, it is used to penetrate into the retina to see structure at the level of the choroids. Scatter can be a source of glare for patients. Peripheral light sources can scatter off scattering bodies in the cornea and lens and end up on the fovea. This scattered light is superimposed onto the image from the object on which the person is fixated. The scattered light causes a reduction in contrast in the image, thus making it more difficult to see. Slide 4 illustrates scatter.⁴

Fluorescence

Molecules can absorb photons of light and move into an excited state. At a later time, they can emit a photon to return to a lower energy state. This process is the foundation for fluorescence. Typically, a photon of a given wavelength is absorbed by the molecule causing a change in the molecular state. As the molecule returns to a resting state, some energy is lost due to vibrational and rotational effects, while the remaining energy is emitted as a photon. The new photon has a longer wavelength than the original photon. Fluorescein dye is routinely used to evaluate corneal integrity and the fit of contact lenses. The dye is illuminated with a blue wavelength of light and it emits or fluoresces in the green.³

Polarization

Polarization deals with the orientation of the electric field in a propagating electromagnetic wave. Unpolarized light has its electric field in random orientations. Conversely, polarized light has its electric field oriented in a single plane. Polaroid material is designed to only transmit light that has its electric field oriented in one direction. If the pass-axis of the Polaroid is lined up with the electric field of incident light, the light is transmitted. If the Polaroid is then rotated 90°, the light is blocked. Light from the sun is unpolarized. On average, the electric field of sunlight is oriented in one direction 50% of the time and oriented 90° away the other 50% of the time. Sunlight incident on Polaroid sunglasses would, therefore, allow only have half of its light to be transmitted. Sunlight reflecting off a shiny object becomes partially polarized in the horizontal direction. By orienting the pass-axis of the Polaroid in the vertical direction, the reflected light is dramatically reduced. Thus, Polaroid sunglasses can dramatically reduce the effects of glare from reflections.⁴

Transmission, Reflection, Absorption and Refraction

As light is incident upon the interface between two media, the light can be transmitted, reflected, or absorbed. Generally, all three happen and just the relative amounts of each vary depending on the material. For visible wavelengths, glass provides high transmission, little absorption, and a small amount of reflection. When looking through a store window, a reflection of yourself is clearly seen. The reflected light corresponds to approximately 4% of the incident light, while the transmitted light is almost 96% (less than 1% of the light is typically absorbed by window glass). A chrome bumper provides nearly 100% reflection, with a small amount of absorption and no transmission. Asphalt absorbs a large portion of the incident light, reflects a small portion of the light (since we see it as a dark shade of gray), and transmits nothing. The amount of light transmitted and reflected from the interface between materials is governed by the angle of incidence of the light and the indices of refraction of the two materials. Generally, significant differences in the indices of refraction cause more light to be reflected. The Purkinje images are an example of light reflected from the interfaces of differing materials. The first Purkinje image is the reflection from the anterior corneal surface. The second Purkinje is the reflection from the cornea-aqueous interface. The third Purkinje is the reflection from the aqueous-lens interface and the fourth Purkinje is the reflection from the lens-vitreous interface. The differences in indices of refraction between the various internal media of the eye are small. Consequently, the second, third, and fourth Purkinje images are dim. The difference between the index of refraction of air and the index of refraction of the cornea is large, leading to a bright reflection in the first Purkinje image.²

Slide 5. Laws of reflection and refraction.

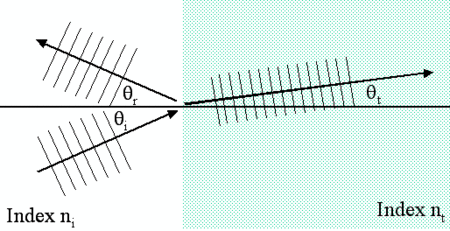
The waves incident on the boundary between two media change direction as a result of interacting with boundary. The laws of reflection and refraction govern these direction changes. The law of reflection indicates that the angle of incidence, q_i, is equal to the angle of reflection, q_r. The angles are measured relative to a line perpendicular to the interface. The law of reflection is analogous to a billiard ball reflecting off a bumper. The law of refraction governs the property of the transmitted wave. The law of refraction, also known as Snell’s law, is given by

n_i sin q_i = n_t sin q_t (1)

where n_i is the index of refraction in the incident media, q_i is the angle of incidence, n_t is the index of refraction of the media the transmitted wave travels into, and q_t is the angle of refraction. Slide 5 illustrates the laws of reflection and refraction. Generally, if the index of refraction of the transmitted media is higher than the incident media, then the wave will bend toward the line perpendicular to the interface (q_t < q_i). Conversely, if the wave is traveling from a higher index to a lower index, the transmitted wave bends away from the perpendicular (q_i < q_t). The optics behind mirrors and lenses is based on these principles. Essentially, lenses are a way of changing the shape of the interface so that the angles of incidence and refraction at various points on the surface cause the wave to converge or diverge. Similarly, curved mirrors are designed to cause the reflected wave to focus or diverge.

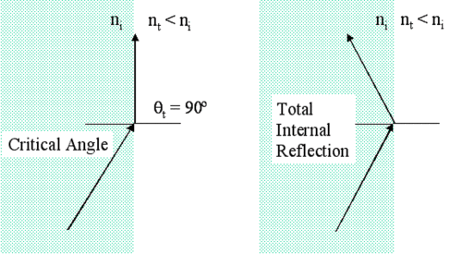
Total Internal Reflection

The maximum value for the angle of refraction, q_t, is 90°. This occurs when the transmitted wave is traveling in the same direction as the interface. In this case, equation 1 for Snell’s law can be written such that

Slide 6. Critical angle and total internal reflection.

Taking the inverse sine of both sides of equation 2 allows the angle of incidence corresponding to this special situation to be found. In the case in which the transmitted wave is traveling in the same direction as the interface, the angle of incidence is called the critical angle. Trigonometry says that the sine of an angle cannot be greater than the unity. In examining equation 2, this trigonometric requirement is only true when n_t < n_i. To get a transmitted wave traveling in the same direction as the interface, the light must be going from a region of higher index to a region of lower index. What happens if the angle of incidence now becomes larger than the critical angle? In this case, total internal reflection occurs. For angles of incidence greater than the critical angle, 100% of the light is reflected from the interface. Slide 6 illustrates the critical angle and total internal reflection. Total internal reflection is used in fiber optics. A high index of refraction core material is sheathed with a lower index material. After injecting light into one end of the fiber, the light undergoes repeated total internal reflection at the core-sheath boundary and remains within the fiber until it comes out the other end. Total internal reflection is also the reason why the angle in the anterior chamber cannot be visualized without the aid of a gonioscopy lens. Light coming from the angle has total internal reflection at the anterior corneal interface. In other words, light leaving the angle tries to go from the higher index cornea to the lower index air, but has an angle of incidence that exceeds the critical angle. A gonioscopy lens is placed in contact with the cornea, so that light travels from the lower index cornea to a higher index glass lens without any total internal reflection. The gonioscopy lens is designed such that when the light reaches the lens-air interface, it is now below the critical angle and can be seen from outside the eye.²

Quantification of Light

Light can be quantified both radiometrically and photometrically. Radiometric measurements are in terms of absolute measurements of energy of electromagnetic radiation without regard to wavelength. Photometric measurements take into account the spectral response of the human visual system and, therefore, should be used only for quantifying visible light. A photon has a certain energy that originated from the energy lost when an excited atom emitted the photon. Thus, the amount of energy in a beam of light is proportional to the number of photons in the beam.

Pulse lasers are typically specified in terms of the number of joules (J, a unit of energy) per pulse. This specification provides the number of photons present in each pulse. Continuous wave lasers, which emit a constant stream of light, are typically rated in terms of watts. A watt (W), which is a unit of power, is the number of Joules per second that the laser emits or, more fundamentally, is proportional to the number of photons the laser emits per second.

Another important concept in radiometry is irradiance. Irradiance is the number of watts per unit area (typically given as W/cm² or W/m²). If all of the photons from a beam of light are concentrated into a small area, then the irradiance is much higher than if the photons are spread over a large area. Sunlight falling onto a piece of paper has much lower irradiance than when the sunlight is collected with a magnifying glass and concentrated to a point. The higher irradiance in the latter case is sufficient to ignite the paper. Safety standards exist for ocular and skin exposure to laser and nonlaser sources and are based on the units of measurement discussed above. Photometry has analogous units of measurement, but now consider the wavelength and our eye’s response to that wavelength in making calculations. Thus, it takes many more photons from the blue or red end of the spectrum to achieve the same photometric effect as a single photon at a green wavelength, since the human spectral response peaks in the green.

The lumen (lm) is analogous to the watt in that it is related to the number of photons a light source radiates in a given second, but the number of photons is weighted by spectral response of the eye. Illuminance, given in terms lm/m² or lux, is analogous to irradiance. It represents the number of spectrally weighted photons striking a given area every second. Radiometry is useful for describing ophthalmic systems where the light interacts with the tissue of the eye, such as in laser photocoagulation and excimer laser refractive surgery. Photometry is useful for analyzing systems in which the eye is the final detector of the light (e.g., designing computer displays or indoor illumination).⁵

References

1. Miller D. Optics and Refraction: A User-Friendly Guide. New York: Gower Medical; 1992.
2. Jenkins FA, White HE. Fundamentals of Optics. New York: McGraw-Hill; 1976.
3. Michaels DD. Visual Optics and Refraction. 2nd ed. St. Louis: Mosby; 1980.
4. Hecht E. Optics. 4th ed. Boston: Addison-Wesley; 2001.
5. Emsley HH. Visual Optics, Vol 2. 5th ed. London: Butterworth; 1952.